Introduction to quantum groups

Notes: Zenodo / local talk 1, talk 2, talk 3, Recordings: Zenodo

Brief introductory seminar series presented to my research group (led by Bruce Bartlett), with a few guests.

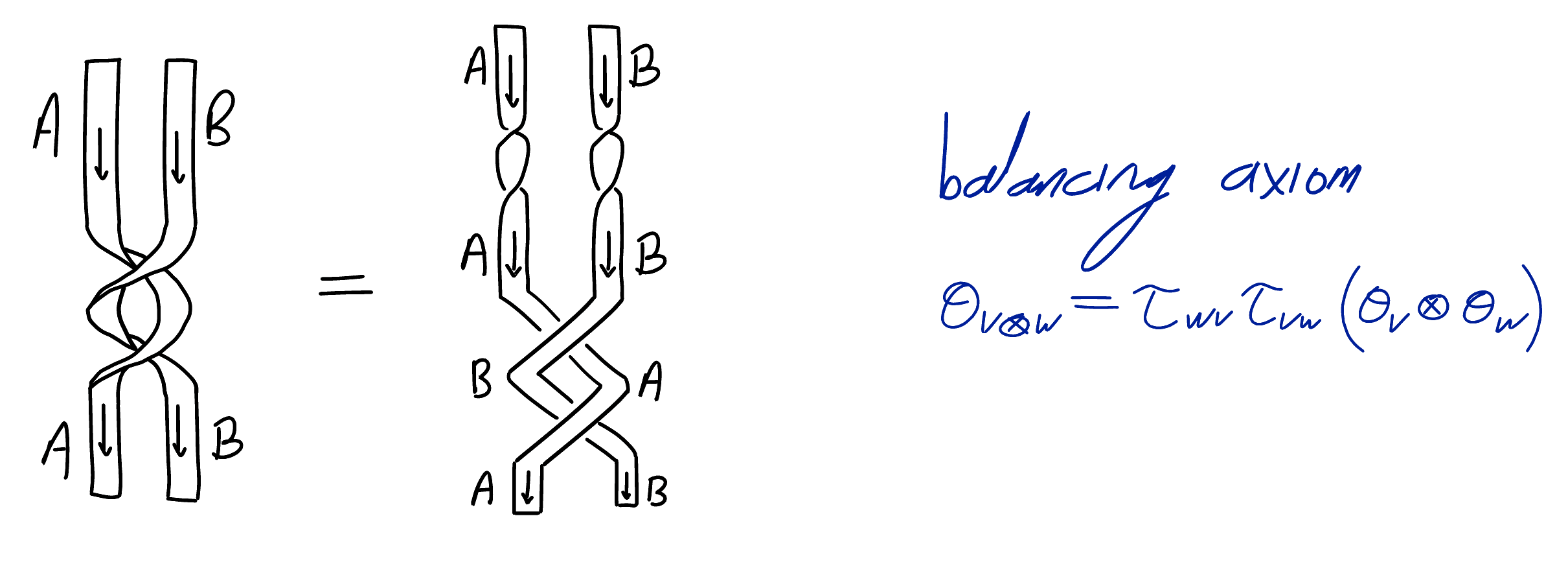
Overview

Our goal is to introduce quantum groups assuming little background, showing a few examples, but focusing mainly on the perspective that quantum groups give rise to link invariants through their representation category.

The presentation is far from exhaustive - this topic is vast, and a thorough treatment requires little short of an entire textbook. That said, my notes include references (in pink) for each section, which should help an interested reader dive deeper. Notable omissions are the Drinfeld quantum double, the construction of quantum $\mathfrak{sl}(2)$, and proofs of several assertions.

Breakdown of talks

1. 29/09/2023

   Notes: Zenodo / local, Recording: Zenodo

   We motivate the Yang-Baxter equation, introduce universal enveloping algebras & Hopf algebras, & see our first examples of quantum groups.

2. 06/10/2023

   Notes: Zenodo / local, Recording: Zenodo

   We see another example of a quantum group, show how braided Hopf algebras give Yang-Baxter equation solutions, & discuss the square of the antipode map in a Hopf algebra; finally, we define ribbon categories.

3. 13/10/2023

   Notes: Zenodo / local, Recording: Zenodo

   We revise graphical calculi for various kinds of category, connect various kinds of Hopf algebra (braided, ribbon, etc) with their categories of representations, & finally show how ribbon Hopf algebras produce the Reshetikhin-Turaev link invariant.

Related references

Quantum groups give a framework in which one can formulate the theory of theta functions. Indeed, one of our main references, Gelca, introduces quantum groups for exactly this purpose. Because of this connection, the material of these talks can be directly linked with my talk on theta functions and knots¹.